Research Papers

A common recipe to improve diffusion models at test-time so that samples score highly against a user-specified reward is to introduce the gradient of the reward into the dynamics of the diffusion itself. This procedure is often ill posed, as user-specified rewards are usually only well defined on the data distribution at the end of generation. While common workarounds to this problem are to use a denoiser to estimate what a sample would have been at the end of generation, we propose a simple solution to this problem by working directly with a flow map. By exploiting a relationship between the flow map and velocity field governing the instantaneous transport, we construct an algorithm, Flow Map Trajectory Tilting (FMTT), which provably performs better ascent on the reward than standard test-time methods involving the gradient of the reward. The approach can be used to either perform exact sampling via importance weighting or principled search that identifies local maximizers of the reward-tilted distribution. We demonstrate the efficacy of our approach against other look-ahead techniques, and show how the flow map enables engagement with complicated reward functions that make possible new forms of image editing, e.g. by interfacing with vision language models.

Masked diffusion models (MDMs) have recently emerged as a promising alternative to autoregressive models over discrete domains. MDMs generate sequences in an any-order, parallel fashion, enabling fast inference and strong performance on non-causal tasks. However, a crucial limitation is that they do not support token insertions and are thus limited to fixed-length generations. To this end, we introduce Flexible Masked Diffusion Models (FlexMDMs), a discrete diffusion paradigm that simultaneously can model sequences of flexible length while provably retaining MDMs' flexibility of any-order inference. Grounded in an extension of the stochastic interpolant framework, FlexMDMs generate sequences by inserting mask tokens and unmasking them. Empirically, we show that FlexMDMs match MDMs in perplexity while modeling length statistics with much higher fidelity. On a synthetic maze planning task, they achieve H60% higher success rate than MDM baselines. Finally, we show pretrained MDMs can easily be retrofitted into FlexMDMs: on 16 H100s, it takes only three days to fine-tune LLaDA-8B into a FlexMDM, achieving superior performance on math (GSM8K, 58%->67%) and code infilling performance (52%->65%).

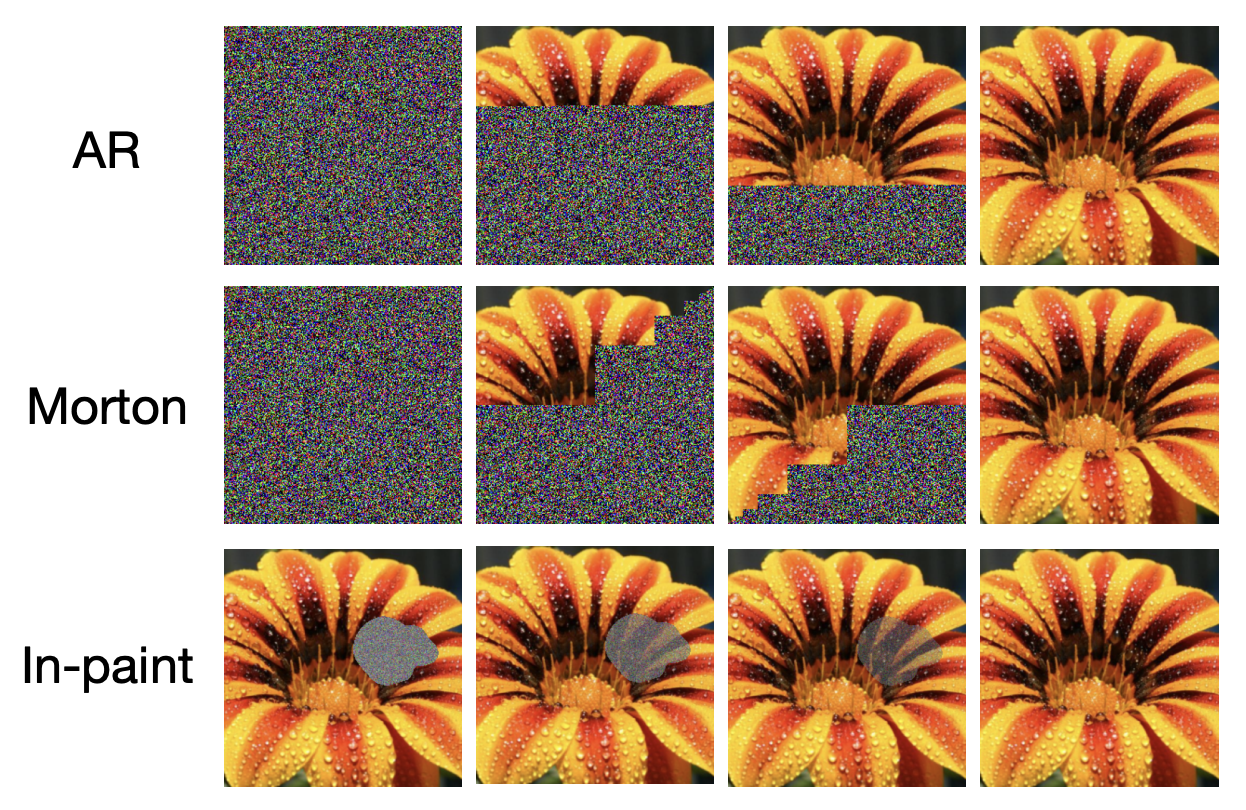
We propose a framework for learning maps between probability distributions that broadly generalizes the time dynamics of flow and diffusion models. To enable this, we generalize stochastic interpolants by replacing the scalar time variable with vectors, matrices, or linear operators, allowing us to bridge probability distributions across multiple dimensional spaces. This approach enables the construction of versatile generative models capable of fulfilling multiple tasks without task-specific training. Our operator-based interpolants not only provide a unifying theoretical perspective for existing generative models but also extend their capabilities. Through numerical experiments, we demonstrate the zero-shot efficacy of our method on conditional generation and inpainting, fine-tuning and posterior sampling, and multiscale modeling, suggesting its potential as a generic task-agnostic alternative to specialized models.

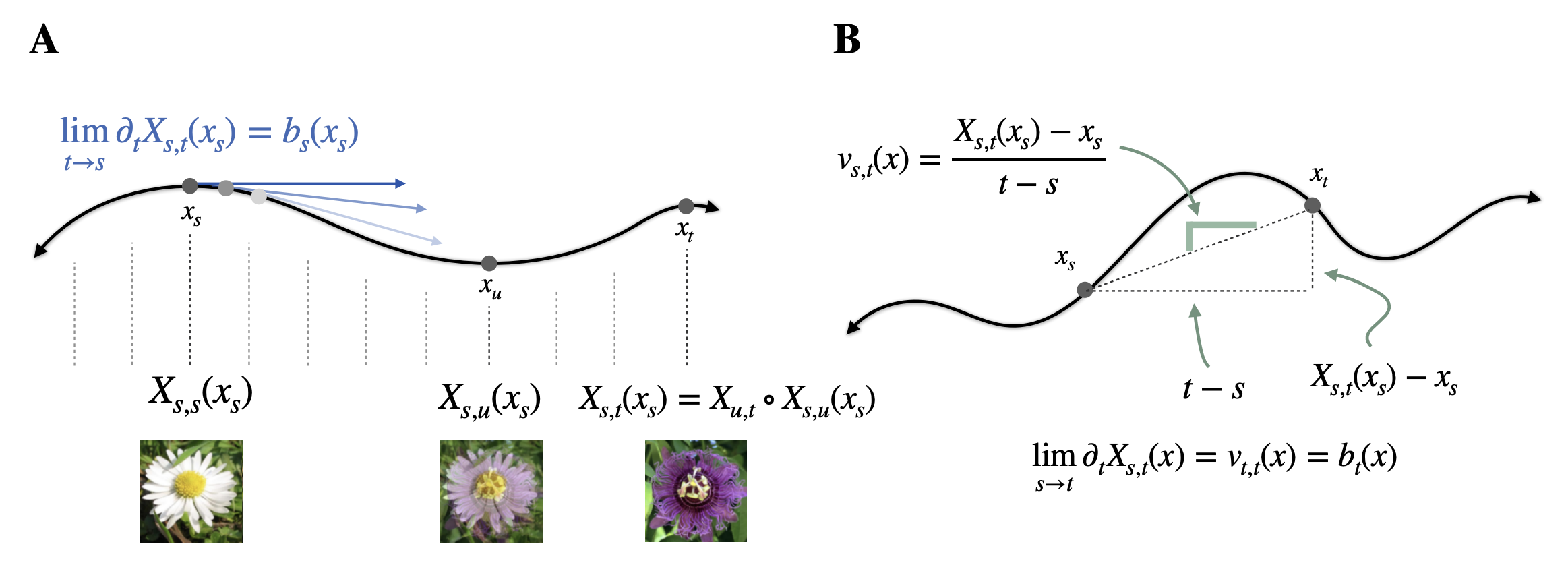
Building on the framework proposed in Boffi et al. (2024), we present a systematic approach for learning flow maps associated with flow and diffusion models. Flow map-based models, commonly known as consistency models, encompass recent efforts to improve the efficiency of generative models based on solutions to differential equations. By exploiting a relationship between the velocity field underlying a continuous-time flow and the instantaneous rate of change of the flow map, we show how to convert existing distillation schemes into direct training algorithms via self-distillation, eliminating the need for pre-trained models. We empirically evaluate several instantiations of our framework, finding that high-dimensional tasks like image synthesis benefit from objective functions that avoid temporal and spatial derivatives of the flow map, while lower-dimensional tasks can benefit from objectives incorporating higher-order derivatives to capture sharp features.

We propose LEAPS, an algorithm to sample from discrete distributions known up to normalization by learning a rate matrix of a continuous-time Markov chain (CTMC). LEAPS can be seen as a continuous-time formulation of annealed importance sampling and sequential Monte Carlo methods, extended so that the variance of the importance weights is offset by the inclusion of the CTMC. To derive these importance weights, we introduce a set of Radon-Nikodym derivatives of CTMCs over their path measures. Because the computation of these weights is intractable with standard neural network parameterizations of rate matrices, we devise a new compact representation for rate matrices via what we call locally equivariant functions. To parameterize them, we introduce a family of locally equivariant multilayer perceptrons, attention layers, and convolutional networks, and provide an approach to make deep networks that preserve the local equivariance. This property allows us to propose a scalable training algorithm for the rate matrix such that the variance of the importance weights associated to the CTMC are minimal. We demonstrate the efficacy of LEAPS on problems in statistical physics.

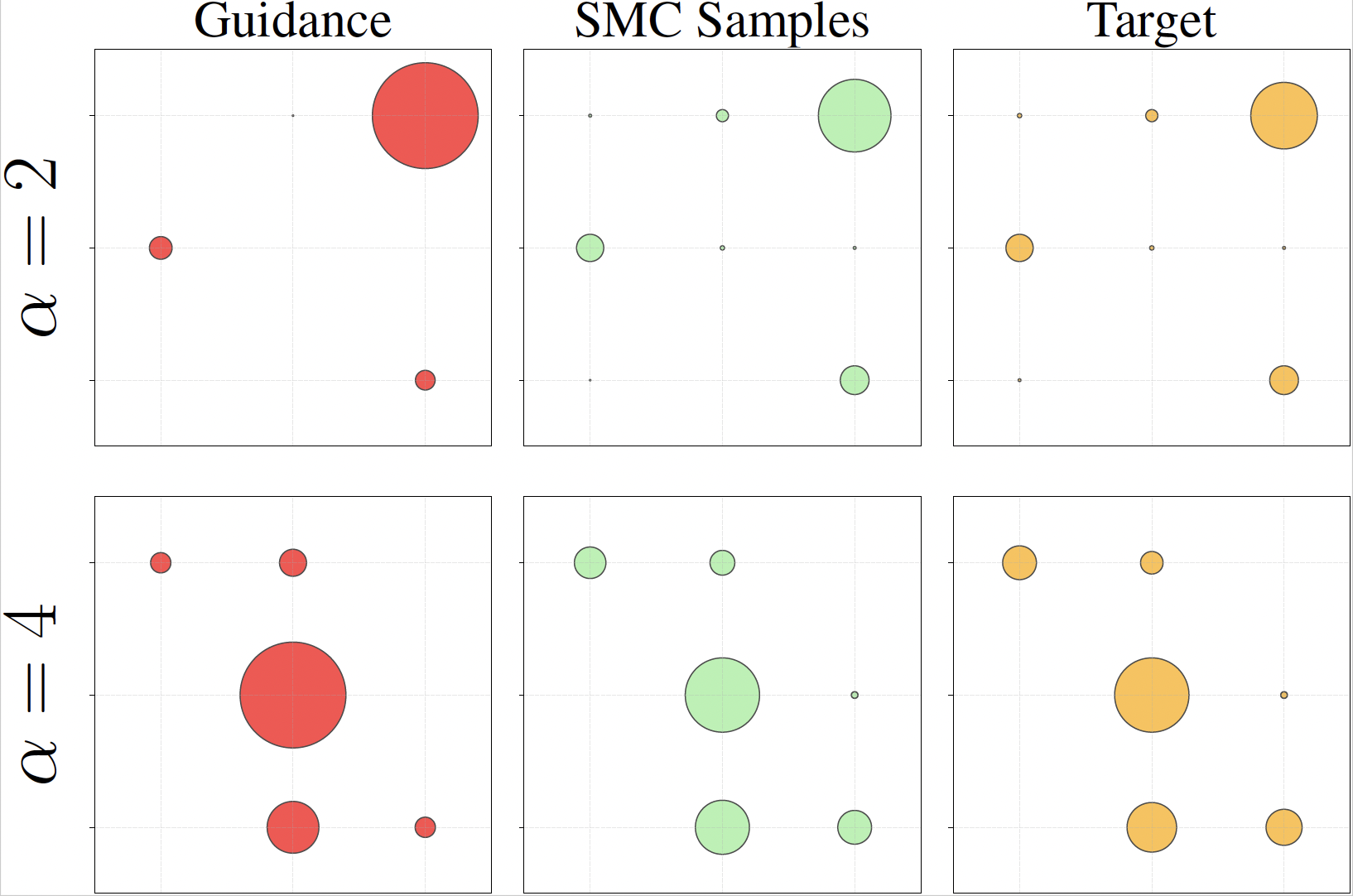
Discrete diffusion models are a class of generative models that produce samples from an approximated data distribution within a discrete state space. Often, there is a need to target specific regions of the data distribution. Current guidance methods aim to sample from a distribution with mass proportional to p0(x0)p(�|x0)^� but fail to achieve this in practice. We introduce a Sequential Monte Carlo algorithm that generates unbiasedly from this target distribution, utilising the learnt unconditional and guided process. We validate our approach on low-dimensional distributions, controlled images and text generations. For text generation, our method provides strong control while maintaining low perplexity compared to guidance-based approaches.

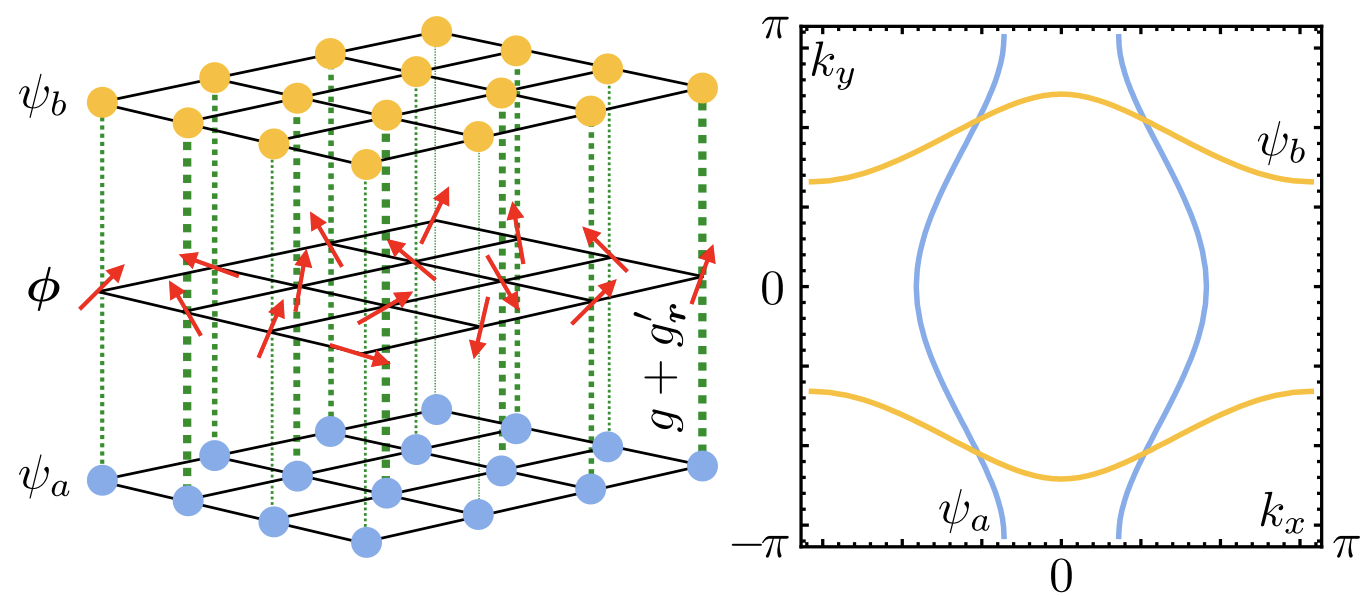
'Strange' metals that do not follow the predictions of Fermi liquid theory are prevalent in materials that feature superconductivity arising from electron interactions. In recent years, it has been hypothesized that spatial randomness in electron interactions must play a crucial role in strange metals for their hallmark linear-in-temperature (T) resistivity to survive down to low temperatures where phonon and Umklapp processes are ineffective, as is observed in experiments. However, a clear picture of how this happens has not yet been provided in a realistic model free from artificial constructions such as large-N limits and replica tricks. We study a realistic model of two-dimensional metals with spatially random antiferromagnetic interactions in a non-perturbative regime, using numerically exact high-performance large-scale hybrid Monte Carlo and exact averages over the quenched spatial randomness. Our simulations reproduce strange metals' key experimental signature of linear-in-T resistivity with a 'planckian' transport scattering rate �tr

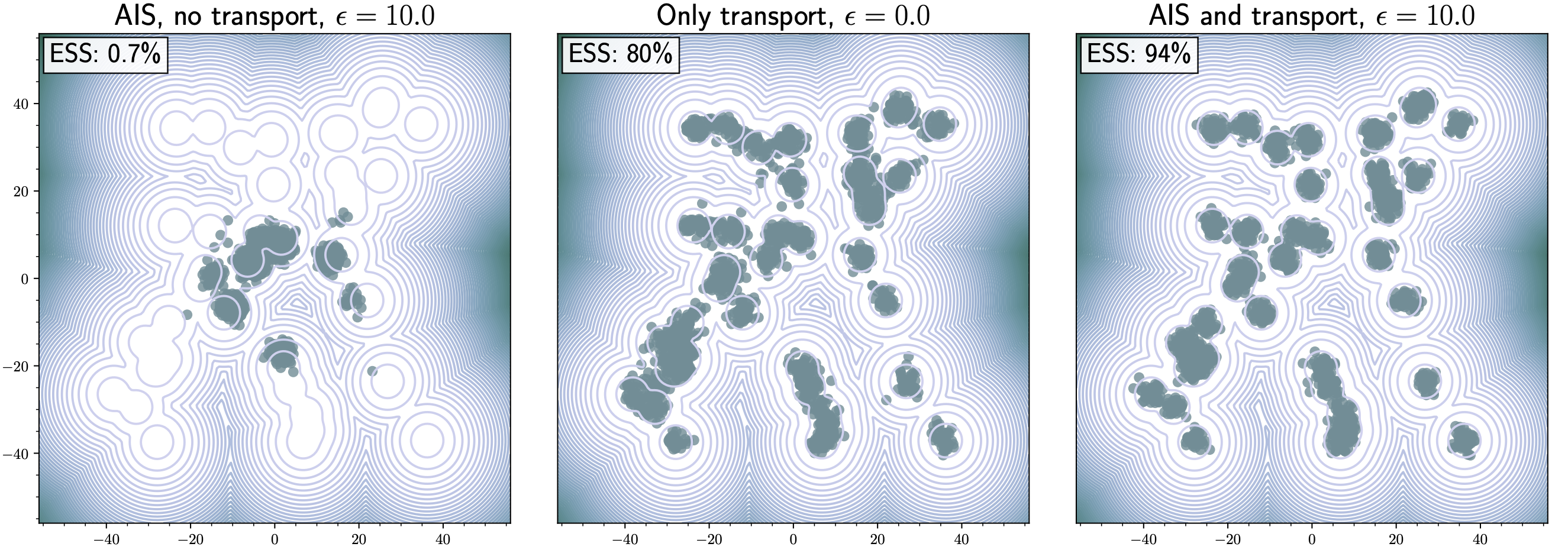
We propose an algorithm, termed the Non-Equilibrium Transport Sampler (NETS), to sample from unnormalized probability distributions. NETS can be viewed as a variant of annealed importance sampling (AIS) based on Jarzynski's equality, in which the stochastic differential equation used to perform the non-equilibrium sampling is augmented with an additional learned drift term that lowers the impact of the unbiasing weights used in AIS. We show that this drift is the minimizer of a variety of objective functions, which can all be estimated in an unbiased fashion without backpropagating through solutions of the stochastic differential equations governing the sampling. We also prove that some these objectives control the Kullback-Leibler divergence of the estimated distribution from its target. NETS is shown to be unbiased and, in addition, has a tunable diffusion coefficient which can be adjusted post-training to maximize the effective sample size.

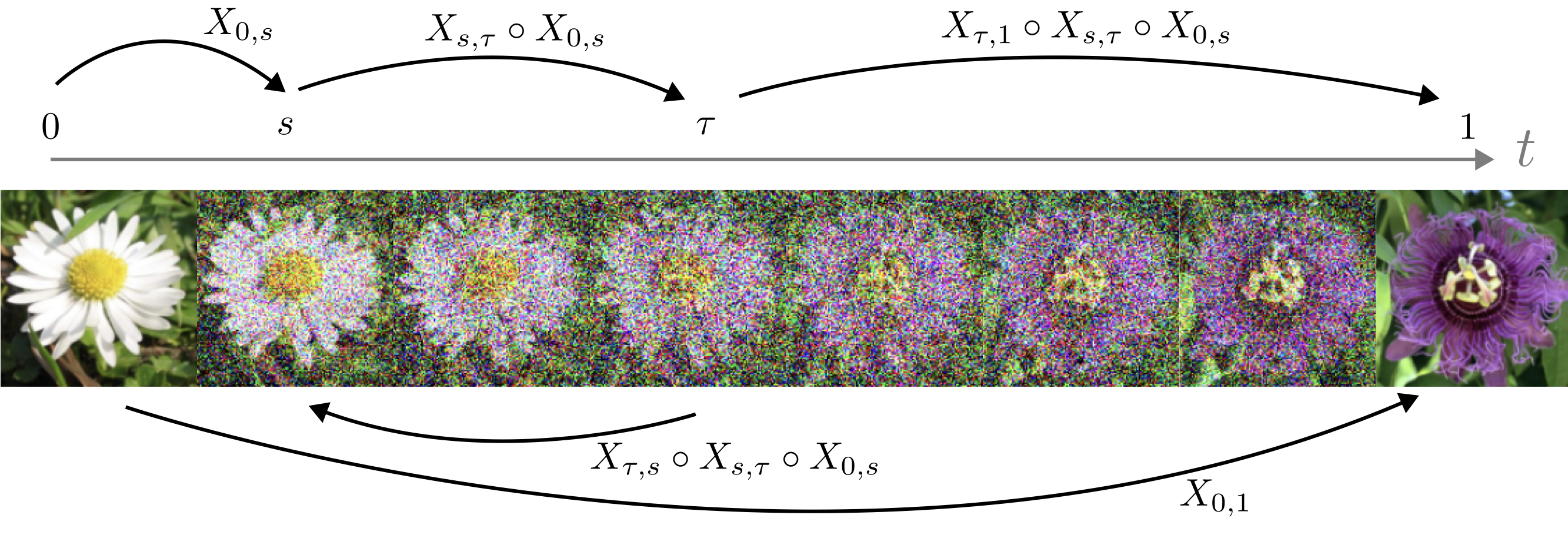
Generative models based on dynamical transport of measure, such as diffusion models, flow matching models, and stochastic interpolants, learn an ordinary or stochastic differential equation whose trajectories push initial conditions from a known base distribution onto the target. While training is cheap, samples are generated via simulation, which is more expensive than one-step models like GANs. To close this gap, we introduce flow map matching -- an algorithm that learns the two-time flow map of an underlying ordinary differential equation. The approach leads to an efficient few-step generative model whose step count can be chosen a-posteriori to smoothly trade off accuracy for computational expense. Leveraging the stochastic interpolant framework, we introduce losses for both direct training of flow maps and distillation from pre-trained (or otherwise known) velocity fields.

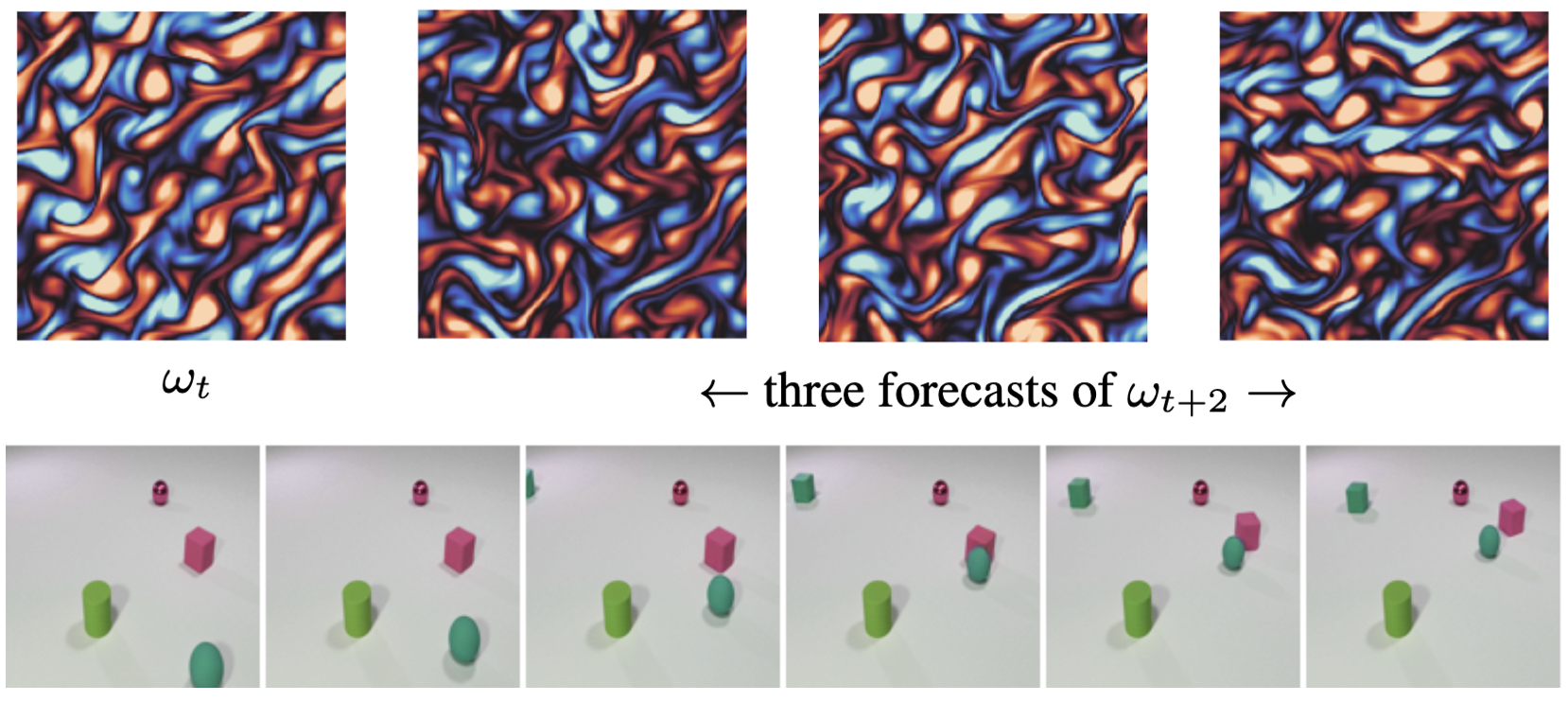
We propose a framework for probabilistic forecasting of dynamical systems based on generative modeling. Given observations of the system state over time, we formulate the forecasting problem as sampling from the conditional distribution of the future system state given its current state. To this end, we leverage the framework of stochastic interpolants, which facilitates the construction of a generative model between an arbitrary base distribution and the target. We design a fictitious, non-physical stochastic dynamics that takes as initial condition the current system state and produces as output a sample from the target conditional distribution in finite time and without bias.

We present Scalable Interpolant Transformers (SiT), a family of generative models built on the backbone of Diffusion Transformers (DiT). The interpolant framework, which allows for connecting two distributions in a more flexible way than standard diffusion models, makes possible a modular study of various design choices impacting generative models built on dynamical transport: using discrete vs. continuous time learning, deciding the objective for the model to learn, choosing the interpolant connecting the distributions, and deploying a deterministic or stochastic sampler. By carefully introducing the above ingredients, SiT surpasses DiT uniformly across model sizes on the conditional ImageNet 256x256 benchmark using the exact same backbone, number of parameters, and GFLOPs.

These lecture notes provide an introduction to recent advances in generative modeling methods based on the dynamical transportation of measures, by means of which samples from a simple base measure are mapped to samples from a target measure of interest. Special emphasis is put on the applications of these methods to Monte-Carlo (MC) sampling techniques, such as importance sampling and Markov Chain Monte-Carlo (MCMC) schemes. In this context, it is shown how the maps can be learned variationally using data generated by MC sampling, and how they can in turn be used to improve such sampling in a positive feedback loop.

Generative models inspired by dynamical transport of measure -- such as flows and diffusions -- construct a continuous-time map between two probability densities. Conventionally, one of these is the target density, only accessible through samples, while the other is taken as a simple base density that is data-agnostic. In this work, using the framework of stochastic interpolants, we formalize how to couple the base and the target densities. This enables us to incorporate information about class labels or continuous embeddings to construct dynamical transport maps that serve as conditional generative models.

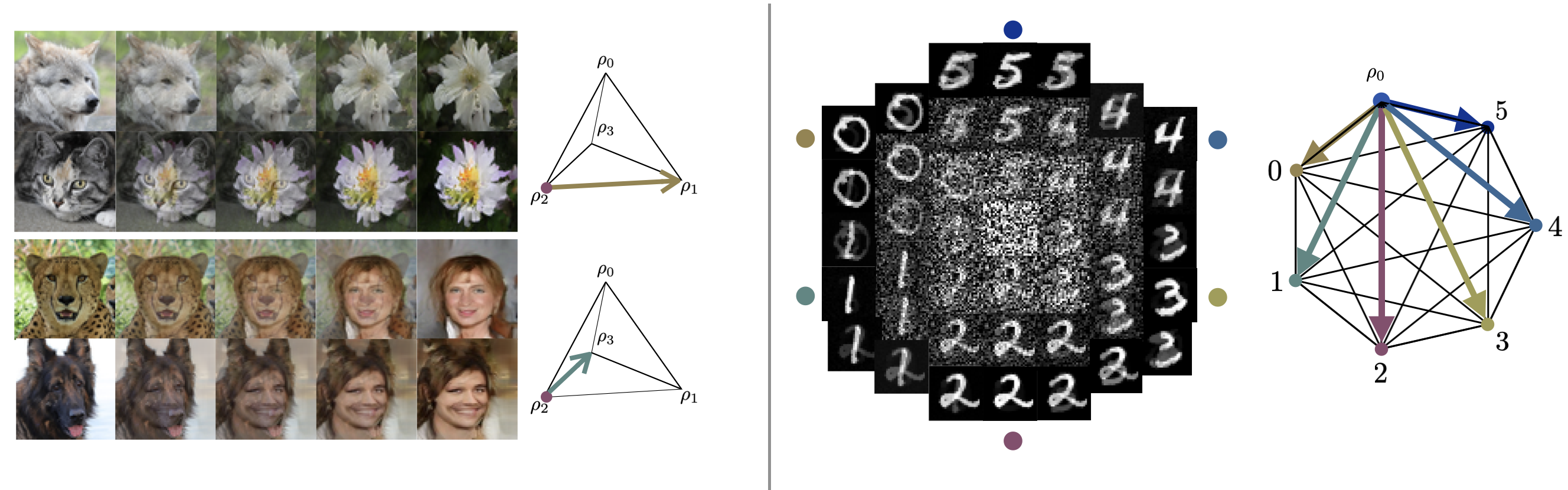
Given a set of K probability densities, we consider the multimarginal generative modeling problem of learning a joint distribution that recovers these densities as marginals. The structure of this joint distribution should identify multi-way correspondences among the prescribed marginals. We formalize an approach to this task within a generalization of the stochastic interpolant framework, leading to efficient learning algorithms built upon dynamical transport of measure. Our generative models are defined by velocity and score fields that can be characterized as the minimizers of simple quadratic objectives, and they are defined on a simplex that generalizes the time variable in the usual dynamical transport framework.

Applications of normalizing flows to the sampling of field configurations in lattice gauge theory have so far been explored almost exclusively in two space-time dimensions. We report new algorithmic developments of gauge-equivariant flow architectures facilitating the generalization to higher-dimensional lattice geometries. Specifically, we discuss masked autoregressive transformations with tractable and unbiased Jacobian determinants, a key ingredient for scalable and asymptotically exact flow-based sampling algorithms. For concreteness, results from a proof-of-principle application to SU(3) lattice gauge theory in four space-time dimensions are reported.

We introduce a class of generative models based on the stochastic interpolant framework proposed in Albergo & Vanden-Eijnden (2023) that unifies flow-based and diffusion-based methods. We first show how to construct a broad class of continuous-time stochastic processes whose time-dependent probability density function bridges two arbitrary densities exactly in finite time. These 'stochastic interpolants' are built by combining data from the two densities with an additional latent variable, and the specific details of the construction can be leveraged to shape the resulting time-dependent density in a flexible way.

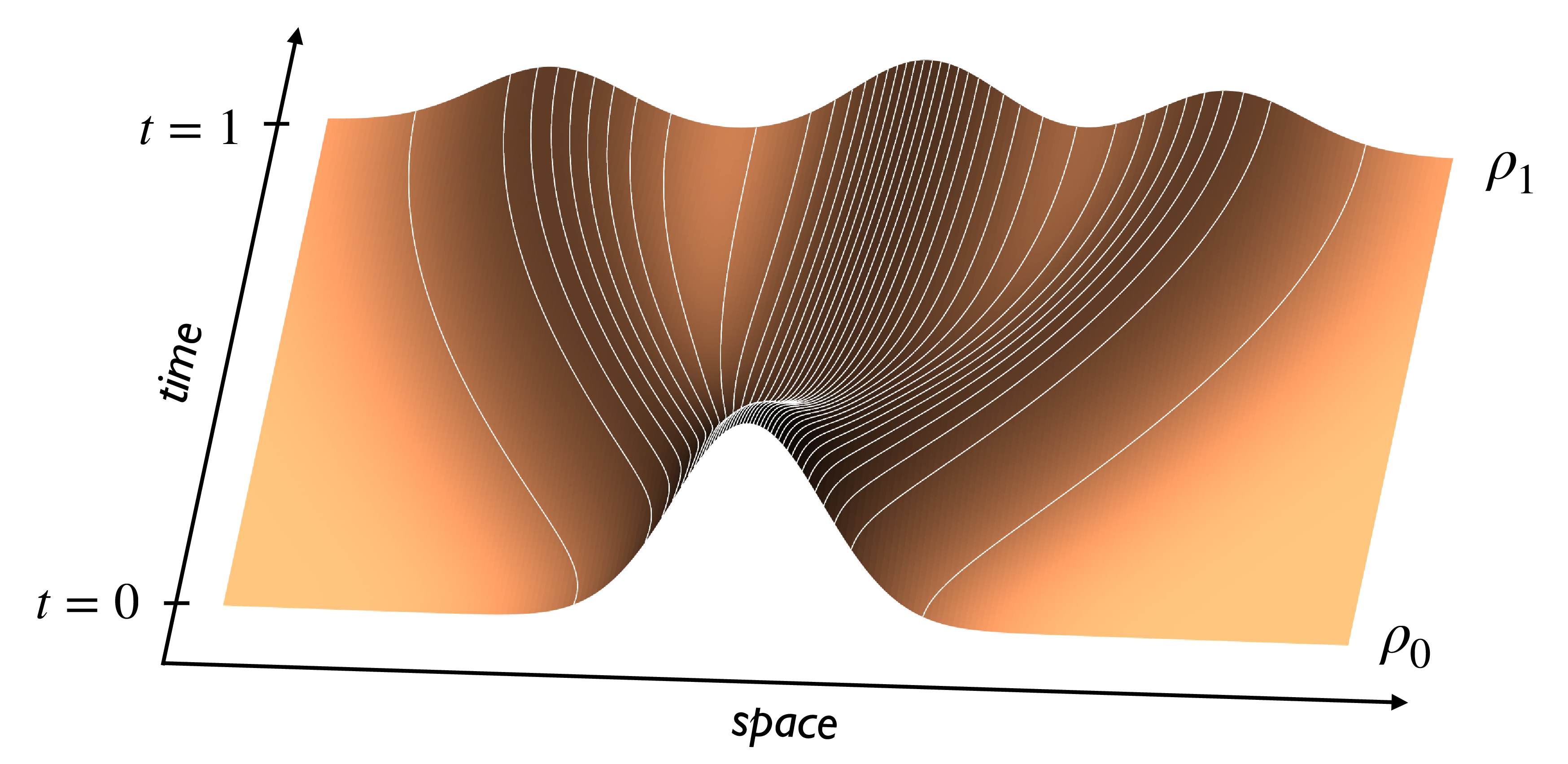
A generative model based on a continuous-time normalizing flow between any pair of base and target probability densities is proposed. The velocity field of this flow is inferred from the probability current of a time-dependent density that interpolates between the base and the target in finite time. Unlike conventional normalizing flow inference methods based the maximum likelihood principle, which require costly backpropagation through ODE solvers, our interpolant approach leads to a simple quadratic loss for the velocity itself which is expressed in terms of expectations that are readily amenable to empirical estimation.

Machine learning methods based on normalizing flows have been shown to address important challenges, such as critical slowing-down and topological freezing, in the sampling of gauge field configurations in simple lattice field theories. A critical question is whether this success will translate to studies of QCD. This Proceedings presents a status update on advances in this area. In particular, it is illustrated how recently developed algorithmic components may be combined to construct flow-based sampling algorithms for QCD in four dimensions.

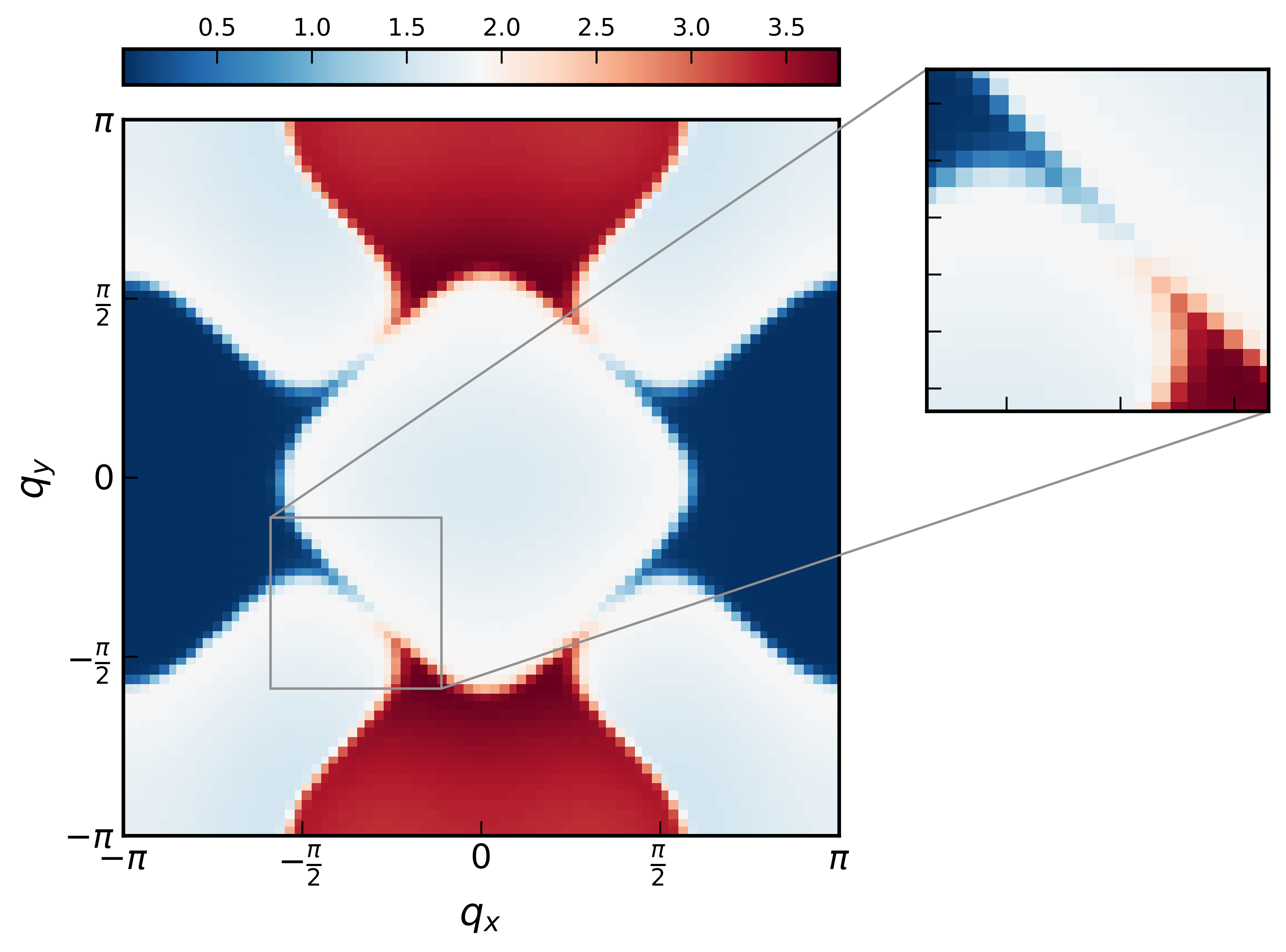
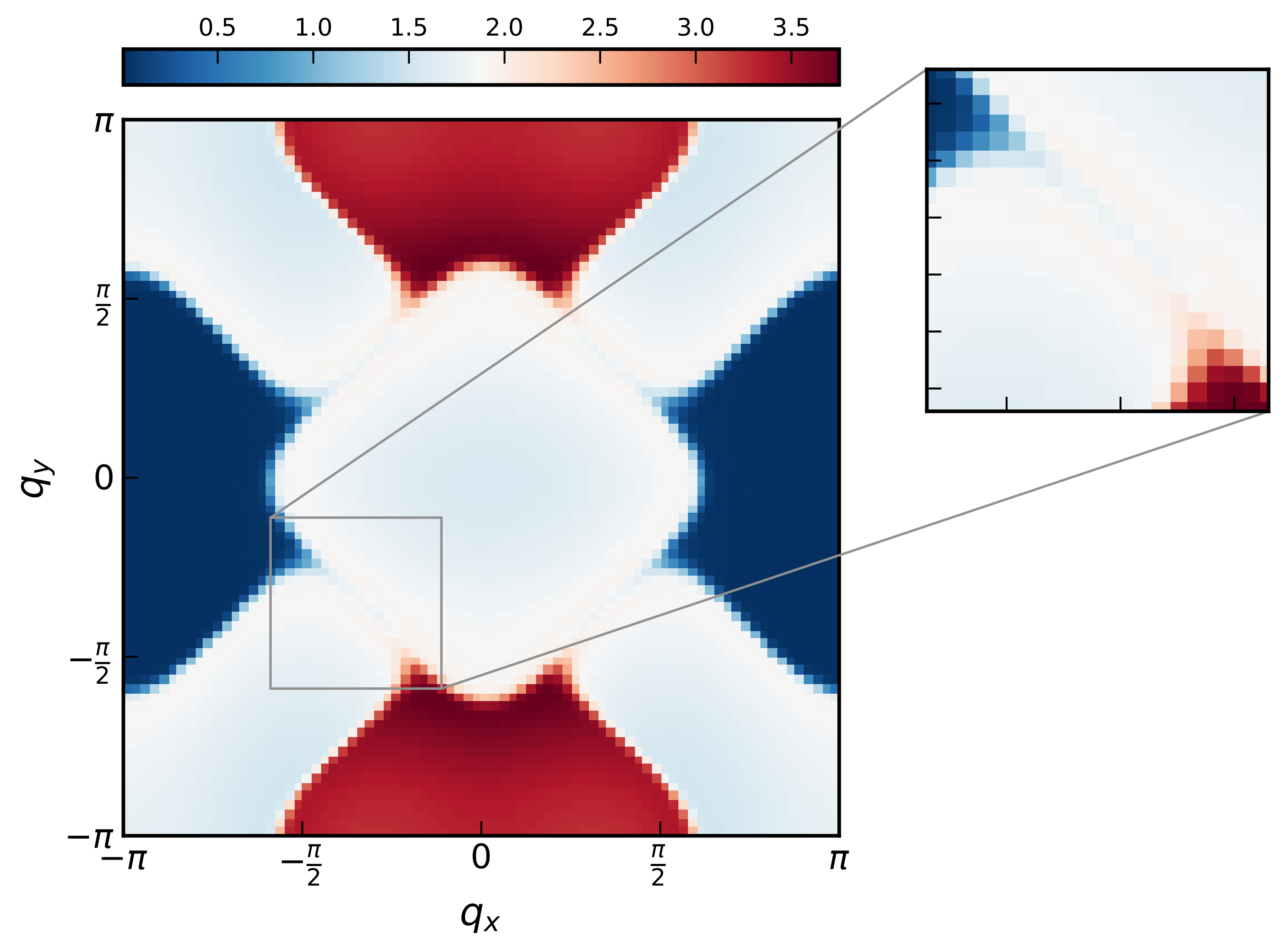
We numerically study the O(3) spin-fermion model, a minimal model of the onset of antiferromagnetic spin-density wave (SDW) order in a two-dimensional metal. We employ a Hybrid Monte Carlo (HMC) algorithm with a novel auto-tuning procedure, which learns the optimal HMC hyperparameters in an initial warmup phase. This allows us to study unprecedentedly large systems, even at criticality. At the quantum critical point, we find a critical scaling of the dynamical spin susceptibility �(�,q) that strongly violates the Hertz-Millis form, which is the first demonstrated instance of such a phenomenon in this model.

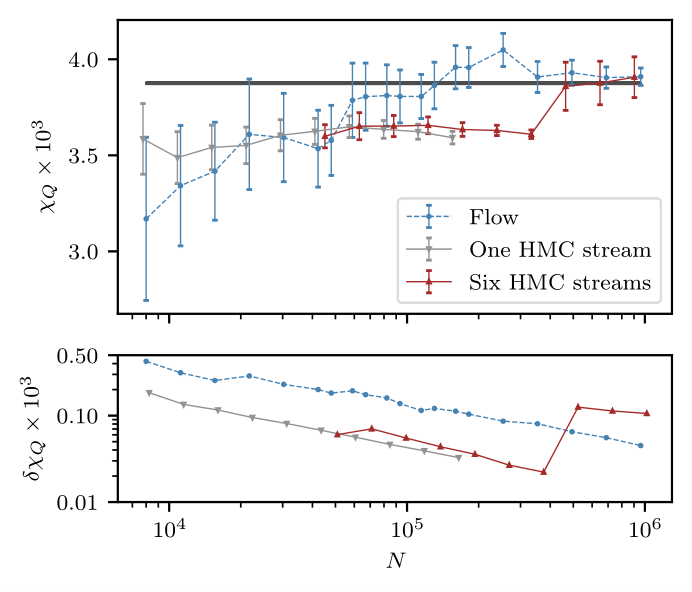
Recent results suggest that flow-based algorithms may provide efficient sampling of field distributions for lattice field theory applications, such as studies of quantum chromodynamics and the Schwinger model. In this work, we provide a numerical demonstration of robust flow-based sampling in the Schwinger model at the critical value of the fermion mass. In contrast, at the same parameters, conventional methods fail to sample all parts of configuration space, leading to severely underestimated uncertainties.

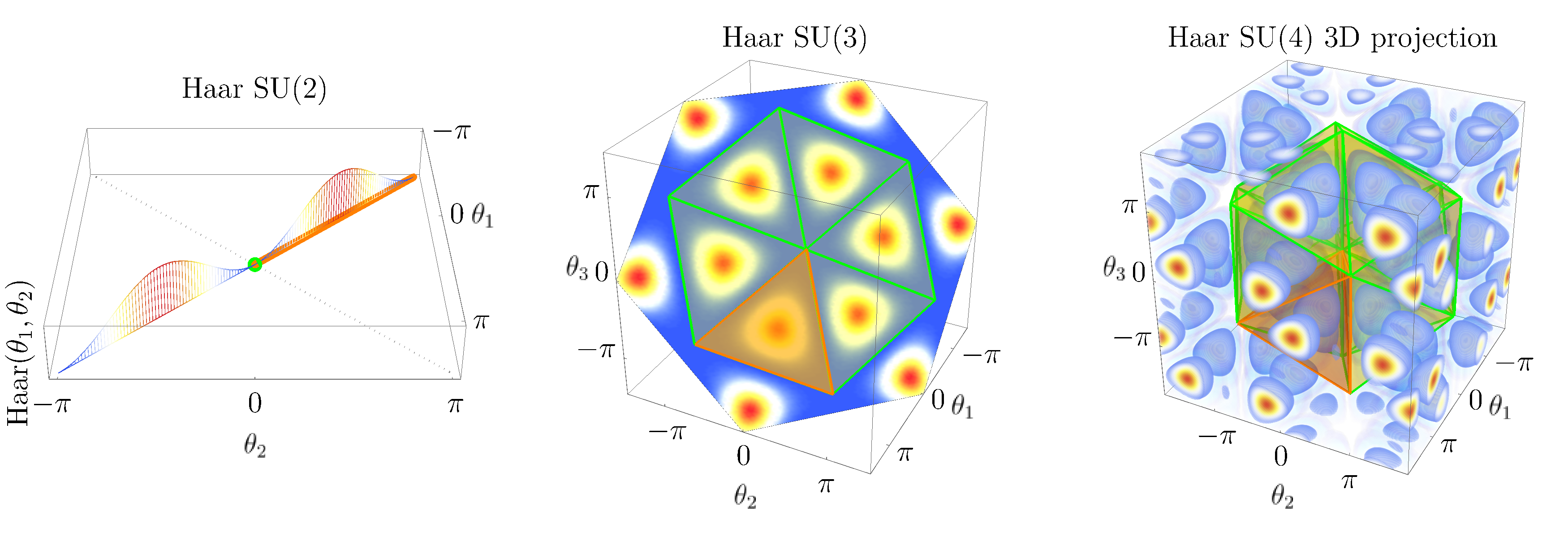
We develop a flow-based sampling algorithm for SU(N) lattice gauge theories that is gauge-invariant by construction. Our key contribution is constructing a class of flows on an SU(N) variable (or on a U(N) variable by a simple alternative) that respect matrix conjugation symmetry. We apply this technique to sample distributions of single SU(N) variables and to construct flow-based samplers for SU(2) and SU(3) lattice gauge theory in two dimensions.

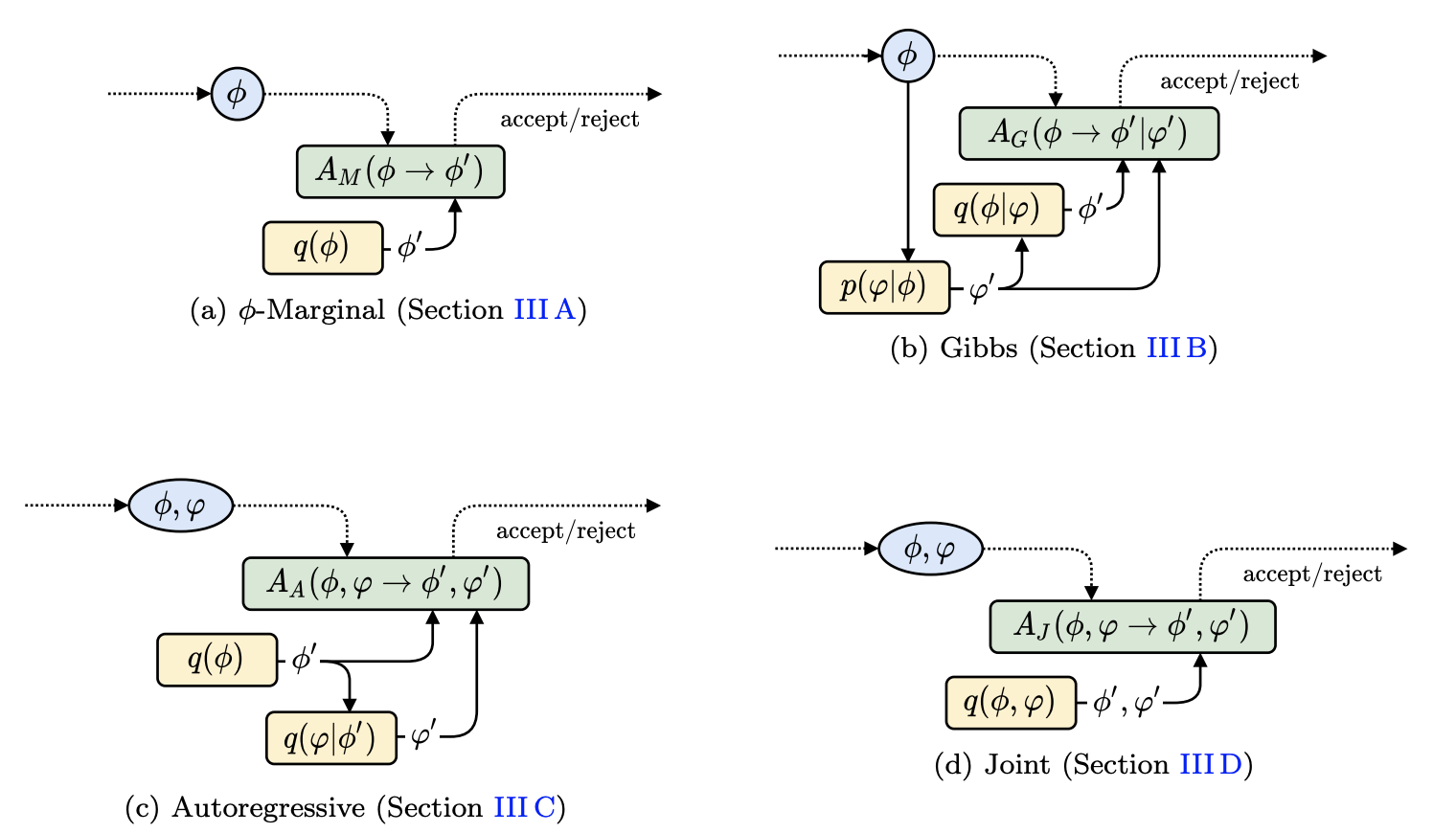
Algorithms based on normalizing flows are emerging as promising machine learning approaches to sampling complicated probability distributions in a way that can be made asymptotically exact. In the context of lattice field theory, proof-of-principle studies have demonstrated the effectiveness of this approach for scalar theories, gauge theories, and statistical systems. This work develops approaches that enable flow-based sampling of theories with dynamical fermions, which is necessary for the technique to be applied to lattice field theory studies of the Standard Model of particle physics and many condensed matter systems.

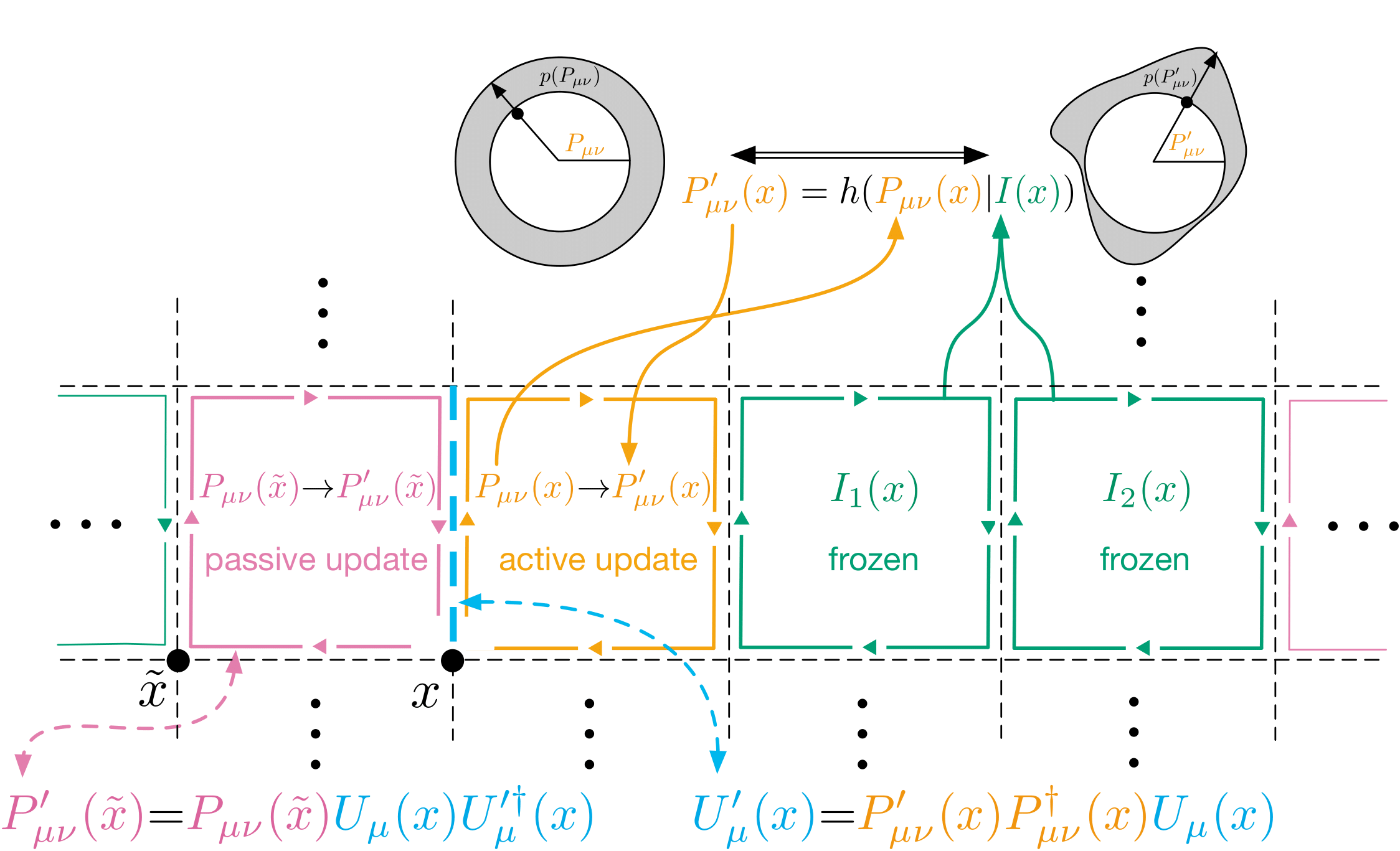
We define a class of machine-learned flow-based sampling algorithms for lattice gauge theories that are gauge invariant by construction. We demonstrate the application of this framework to U(1) gauge theory in two spacetime dimensions, and find that, at small bare coupling, the approach is orders of magnitude more efficient at sampling topological quantities than more traditional sampling procedures such as hybrid Monte Carlo and heat bath.

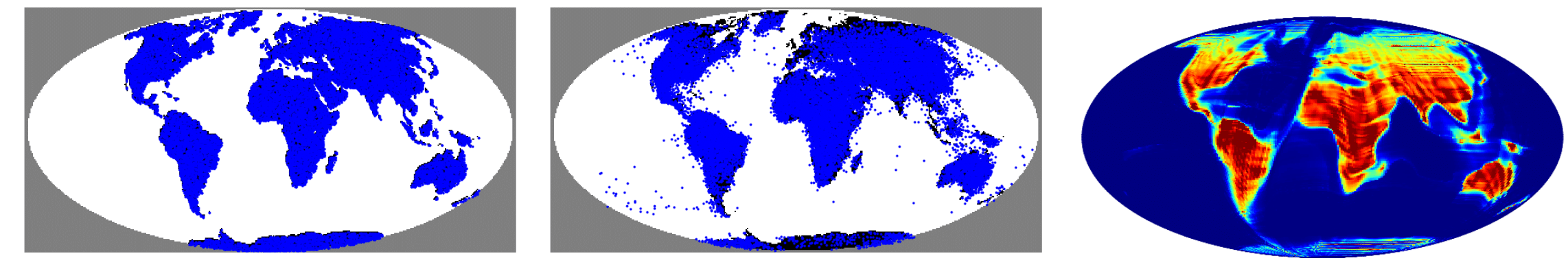
Normalizing flows are a powerful tool for building expressive distributions in high dimensions. So far, most of the literature has concentrated on learning flows on Euclidean spaces. Some problems however, such as those involving angles, are defined on spaces with more complex geometries, such as tori or spheres. In this paper, we propose and compare expressive and numerically stable flows on such spaces. Our flows are built recursively on the dimension of the space, starting from flows on circles, closed intervals or spheres.

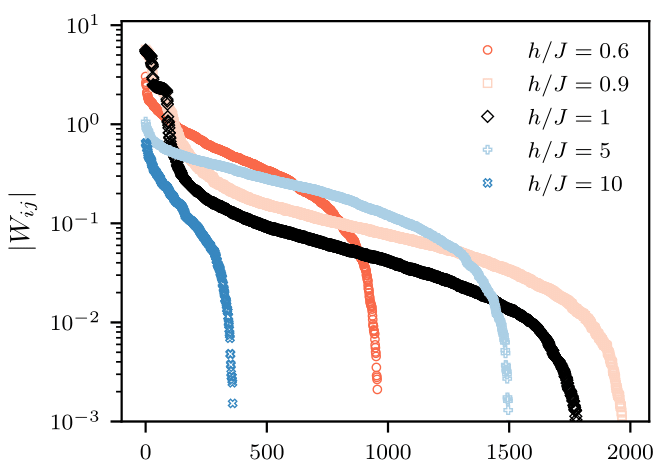
Generative modeling with machine learning has provided a new perspective on the data-driven task of reconstructing quantum states from a set of qubit measurements. As increasingly large experimental quantum devices are built in laboratories, the question of how these machine learning techniques scale with the number of qubits is becoming crucial. We empirically study the scaling of restricted Boltzmann machines (RBMs) applied to reconstruct ground-state wavefunctions of the one-dimensional transverse-field Ising model from projective measurement data.

A Markov chain update scheme using a machine-learned flow-based generative model is proposed for Monte Carlo sampling in lattice field theories. The generative model may be optimized (trained) to produce samples from a distribution approximating the desired Boltzmann distribution determined by the lattice action of the theory being studied. Training the model systematically improves autocorrelation times in the Markov chain, even in regions of parameter space where standard Markov chain Monte Carlo algorithms exhibit critical slowing down in producing decorrelated updates. Moreover, the model may be trained without existing samples from the desired distribution.